|
Consider any of these inequalities:
A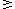 B ,
A B ,
A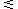 B ,
A > B , A <
B. B ,
A > B , A <
B. |
|
For each such inequality,
it's ASSOCIATED EQUATION is A = B. |
|
We will abbreviate the phrase
"ASSOCIATED EQUATION" to
"AE". |
|
|
|
The AE for the inequality "3x - 2y 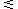 5"
is "3x - 2y = 5" . 5"
is "3x - 2y = 5" . |
|
|
|
Let A and B be algebraic expressions
involving the variables x, y, or others.
The graph of the equation
A = B divides up space into pieces; |
|
within each piece, |
either [1] ALL points satisfy A > B , or
or [2] ALL points satisfy A < B. |
|
|
|
|
|
In our situations, A and B will usually involve the
two variables "x" and "y", the AE's will have
straight-line graphs, and therefore the pieces into
which A = B divides space will be "half-planes",
that is, the set of points on one side of a
straight line. |
|
|
|
To graph an inequality
(such as A 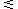 B), first
graph it's AE. Then test each piece into which
that graph divides space (with "TEST POINTS":
red points in figure
below). Choose or mark those pieces whose test
points satisfy the given inequality (in our
example, A B), first
graph it's AE. Then test each piece into which
that graph divides space (with "TEST POINTS":
red points in figure
below). Choose or mark those pieces whose test
points satisfy the given inequality (in our
example, A 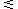 B). Thus, in
the examples below, the origin ( 0 , 0 ) makes a
good test point, for which the stated inequality
" x 2 + y 2 B). Thus, in
the examples below, the origin ( 0 , 0 ) makes a
good test point, for which the stated inequality
" x 2 + y 2 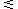 1 "
is TRUE. 1 "
is TRUE. |
|
|
MARKING YOUR GRAPH
ARROWS are used when marking several overlapping regions. Thus,
the graph at the right marks the interior of the circle with
center (0,0) and radius 1, which solves the inequality
" x2 + y2 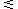 1 ". 1 ". |
|
| |
The test points are C = (0,0) and
D = (2,0): C
satisfies " x2 + y2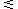 1"
since 1"
since
" 02 + 02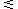 1".
On the other hand, D fails to satisfy
" x2 + y2 1".
On the other hand, D fails to satisfy
" x2 + y2 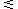 1 ", because
" 22 + 02 = 4 > 1 ". Thus, using Fact 3
above, the solution set for our inequality is the set of
points on the same side of the circle as is
C. 1 ", because
" 22 + 02 = 4 > 1 ". Thus, using Fact 3
above, the solution set for our inequality is the set of
points on the same side of the circle as is
C. |
|
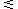 1 ".
1 ".